1、 Definition of the Composite Index

1. Overview

The alloy mixing index is a research and development based on the liquid mixing model of sports powder.

The liquid mixing model of moving powder describes the movement trend of various powders in dynamic mixing. The stronger the trend of "upward floating" and "downward" separation movement, the more difficult it is to mix uniformly.

The Jin He Mixing Index quantitatively describes the strength of this movement trend using mathematical methods, becoming the basis for guiding mixing work. Therefore, mastering the calculation and usage method of the alloy mixing index can easily control the smooth progress of powder mixing.

2. Setting of powder characteristic values

Each type of powder can be characterized by multiplying its specific gravity by its average particle size.

Represented as: B × D

           Where: B=specific gravity of powder

                       D=average particle size of powder

3. Setting of the average value of the characteristics of the powder to be mixed

Let the average characteristic value of the batch of powder to be mixed be H, and let Ki be the proportion (percentage) of the i-th type of powder in the batch of powder to be mixed. The larger the proportion of powder, the greater the impact on the average characteristic value of the batch of powder to be mixed.

      According to the above guiding ideology, the average value of the characteristics can be represented by the following equation:              

        H = B1×D1×K1+…+Bi×Di×Ki+…+Bn×Dn×Kn

        Summarized as:

Where: Bi=specific gravity of the i-th type of powder;

Di=particle size of the i-th type of powder;

Ki=percentage of i-th powder.

I=1~n

That is to say, the average characteristic value of this batch of powder to be mixed is equal to the sum of the product of the specific gravity of each powder multiplied by the average particle size of the powder and then multiplied by its proportion in the batch of powder to be mixed.

4. Definition of the Composite Index

Definition: The alloy mixing index of a specified powder is the ratio of the characteristic value of the powder to the average characteristic value of the batch of powder to be mixed.

Therefore, the following formula represents:

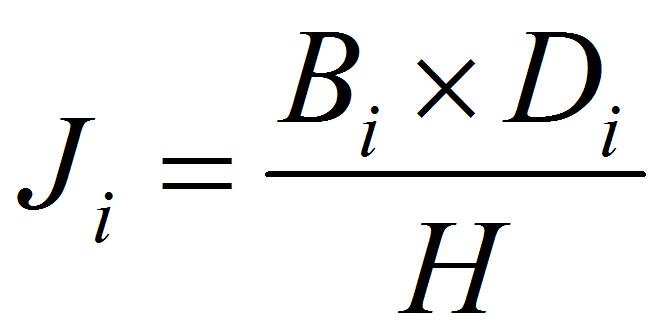
wherein：H = B1×D1×K1+…+Bi×Di×Ki+…+Bn×Dn×Kn                    

In the formula, B1, D1, and K1 represent the specific gravity, average particle size, and percentage of the first type of powder;

Bi, Di, and Ki represent the specific gravity, average particle size, and percentage of the i-th type of powder;

N represents the number of powder species involved in mixing;

H represents the average characteristic value of the batch of powder to be mixed;

Ji represents the alloy mixing index of the i-th type of powder;

When i=1~n, the alloy mixing index J1~Jn of each powder can be calculated separately.

5. Example Calculation of the Composite Index

For example, there are three types of powder to be mixed, and the first step is to measure the quantifiable physical indicators of each type of powder: specific gravity, average particle size, and proportion in the batch of powder to be mixed as follows:

The first type of powder B1=1.1 D1=0.01mm K1=30%

The second type of powder B2=0.9 D2=0.015mm K2=60%

The third type of powder B3=2.1 D3=0.03mm K3=10%

Step 2: Calculate the average characteristic value:

 H =1.1×0.01×30%+0.9×0.015×60%+2.1×0.03×10%

                 =0.0033+0.0081+0.0063

                 =0.0177

        Step3：to calculate the alloy mixing index of the three powders:

               J1=1.1×0.01÷0.0177≈0.62

               J2=0.9×0.015÷0.0177≈0.76

               J3=2.1×0.03÷0.0177≈3.56

2、 Interpretation and Application

1. Interpretation of Metal Composite Blending

1) Each powder in the specified batch of powder to be mixed has a corresponding alloy mixing index

Once the powder to be mixed is determined, the corresponding alloy mixing index for each powder can be calculated using the above formula. The larger the alloy mixing index, the larger the particle size or specific gravity of the powder. The smaller the alloy mixing index, the smaller the particle size or specific gravity of the powder.

2) The value of the alloy mixing index of a powder is related to its own characteristics and also to the characteristics of other powders to be mixed together

If the index of a certain powder is changed, it will affect the alloy mixing index of each other powder, and reducing or increasing the powder will also change the alloy mixing index of each other powder.

2. The practical significance of the Jin He Mixed Index

1) The significance of the numerical value of the composite index

① The value of the alloy mixing index is equal to 1, indicating that the specific gravity and average particle size of the powder are basically consistent with the average value of the overall powder to be mixed, indicating that the powder is easy to participate in mixing;

② If the value of the alloy index is less than 1, it indicates that the powder is lighter or finer compared to the overall average of the powder to be mixed. If its value is below 0.3, it is difficult to mix evenly.

③ If the value of the alloy index is greater than 1, it indicates that the powder is relatively heavy or has a larger particle size compared to the overall average of the powder to be mixed. If its value is above 3.0, it is difficult to mix evenly when participating in the mixing. Usually, we can use the method of refining the average particle size of the powder to reduce its alloy mixing index value, thereby making mixing more uniform and easier.

2) Quantitative analysis of the difficulty of mixing processes using the ratio of the size of the alloy mixing index

Calculate the alloy mixing index of each type of powder to be mixed using the aforementioned formula.

① Select the maximum and minimum alloy mixing indices, and calculate their ratio Z:

Among them, JMAX is the largest alloy mixing index value in the entire batch of powders, and JMIN is the smallest alloy mixing index value.

②  Use the differentiation ratio z to determine the difficulty of the mixing process:

Through a large number of experiments, the following conclusions have been drawn: a ratio z greater than 3 indicates a significant difference in the characteristic values of the two powders, and the difficulty of uniform mixing begins to increase; If the ratio z is greater than 6, it indicates that the characteristic values of the two powders differ greatly, and uniform mixing is a difficult task to achieve.

3) The significance of the Jin He Mixed Index

The size of the difference between the composite mixing indices is the key to the application of the indices. The greater the difference, the closer the physical indicators between the powders are, and it belongs to homogeneous mixing. Powder mixing is easy to achieve the goal of uniformity. On the contrary, the larger the difference in the alloy mixing index, the more differentiated the powder mixing exists, and there are difficulties in mixing light and heavy powders between the powders, making it difficult to mix. Therefore, we can use the alloy mixing index to guide the smooth progress of the powder mixing process.

The Jin He Mixing Index is a theory developed by our company's designer team through years of experience in powder mixing, scientific summary, and independent research.

The introduction of the alloy mixing index aims to quantitatively describe the difficulty level of the mixing process, which provides a very effective and easy to master scientific guidance method for powder mixing work, filling a gap in the theoretical field of powder mixing technology in the domestic and foreign industry.

3. Application of the Composite Index

The Jin He Mixed Index has great practical significance in guiding mixing work.

When the differentiation ratio z of the composite index is within 3, it indicates that the mixing task is easy to complete, and ordinary mixing equipment can be used to implement the mixing.

When the differentiation ratio z of the composite index is greater than 3 or even reaches 10 or above, the difficulty of mixing gradually increases or even makes it difficult to achieve the goal of uniform mixing. The following methods can be used to address this situation:

1) Grinding the larger and heavier particles in the powder reduces the differential ratio z of the index, achieving the goal of easy and uniform mixing.

2) Using a mixer with forced mixing function to solve the problem, such as dual motion ® Mixer. Dual motion ® The rolling and gripping capacity of the mixing machine's bucket can suppress the "upward" and "downward" trends of the powder, thereby achieving the goal of forced mixing uniformity.

4. Application examples

1) Calculate before processing:

Taking the three aforementioned powders as examples, the corresponding alloy mixing index has been calculated:

The first powder B1=1.1 D1=0.01mm J1 ≈ 0.62

The second type of powder B2=0.9 D2=0.015mm J2 ≈ 0.76

The third type of powder B3=2.1 D3=0.03mm J3 ≈ 3.56

Average characteristic value: H=0.0177

Select the maximum and minimum values of the composite index, and calculate the size ratio of their composite index:

Yes: JMAX=J3 ≈ 3.56 JMIN=J1 ≈ 0.62

So the ratio is: Z=J3/J1 ≈ 5.74

2) Analysis: Due to the significant difference in the alloy mixing index between the third powder and the first powder, with a ratio exceeding 5, mixing is difficult. Analyzing the reasons, it can be concluded that the significant difference in the average characteristics between the third type of powder and the overall powder to be mixed is the main reason for the difficulty in mixing. Suggest refining the third type of powder. Because the first and second powders have similar metallurgical mixing indices, while the third powder has much higher metallurgical mixing indices. At the same time, the first and second powders are small and light powders that cannot be coarsened. Therefore, we can only grind the third powder to make its metallurgical mixing indices close to each other, thus achieving the goal of convenient and uniform mixing!

3) After grinding the third powder:

Obtain the characteristic value change of the third powder:

          a1       B1=1.1      D1=0.01mm     K1=30%

          b1       B2=0.9      D2=0.015mm    K2=60%

          c1       B3=1.2      D3=0.016mm    K3=10%

重新计算特性平均值：

         H = 1.1×0.01×30%+0.9×0.015×60%+1.2×0.016×10%

            ≈0.0033+0.0081+0.0019

            ≈0.013

Recalculate the alloy mixing index for each powder:

         J1=1.1×0.01÷0.013≈0.85

         J2=0.9×0.015÷0.013≈1.04

         J3=1.2×0.016÷0.013≈1.48

Select the maximum and minimum composite index, and calculate the ratio Z: Z=JMAX/JMIN=J3/J1 ≈ 1.74

Through processing, it can be seen that the difference in the alloy mixing index of the three powders is already very small, with a ratio Z less than 2. This indicates that the third powder has similar characteristics to the other two powders, and under such conditions, it is easy to achieve the goal of uniform mixing. This effectively utilizes the alloy mixing index to guide the smooth progress of the mixing process!

5. Summary

It can be concluded that the Jin He Mixed Index has three major advantages:

1) Quantify the difficulty of various powder mixing tasks.

2) Accurately identify individuals who are difficult to mix evenly among various powders involved in mixing, facilitating targeted work guidance for the smooth progress of mixing work.

3) The simple detection and calculation of the alloy mixing index can guide the implementation of the mixing process, saving a lot of manpower, material resources, and time losses.